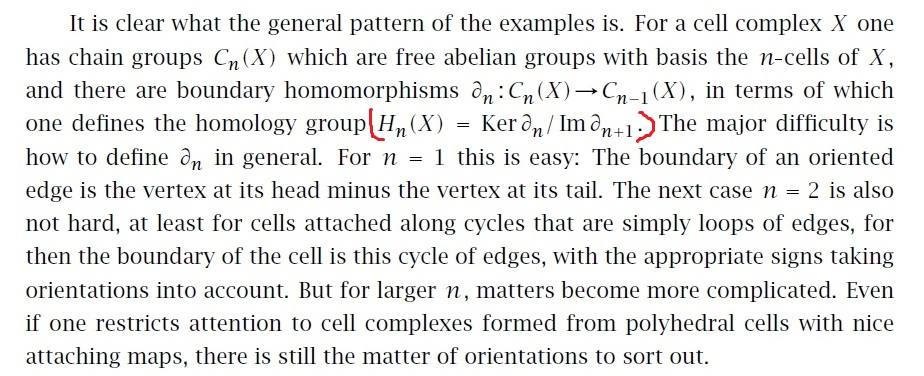
Now to speak my own language:
I’m \partial_{n+1} is the group consisting of all the n-dimensional chains that are boundaries of (collections of) higher-dimensional cells. Ker \partial_n consists of all the n-dimensional chains whose boundaries are empty.
Since the boundary of a boundary is always empty, everything in Im is in Ker. But not everything in Ker is in Im: some figures have no boundary, but due to the fact that the underlying space (i.e. the geometric realization of the cell complex) is twisted in some way, they may not be, in themselves, boundaries of anything (e.g. a circle around the middle of a Moebius strip). Using the quotient by Im gets rid of those chains which are boundary-less for necessary reasons (being boundaries themselves) and leaves only the group that captures this remaining part. This is why it’s a measure of the topology of the space.
This is a decent class hypothesis book:
http://www.math.jhu.edu/~eriehl/context.pdf
As inspiration, I concur that variable based math is great. There are loads of variable based math books out there. I don’t think I have a specific top choice. Familiarize yourself with the meanings of gatherings and gathering activities, rings, and modules would be my suggestion.
Steve Awodey’s book “Category Theory” is aimed at computer scientists and logicians, so for this reason it doesn’t require an algebra background. I would say it’s your best bet.
This book is a text and reference book on Category Theory, a branch of abstract algebra. … It provides full proofs of all the important propositions and theorems, and aims to make the basic ideas, theorems, and methods of Category Theory understandable.